Do you ever feel like math is a secret language, filled with symbols and equations that seem to have a life of their own? It's a world where numbers dance, and relationships are revealed through the elegant simplicity of mathematical statements.
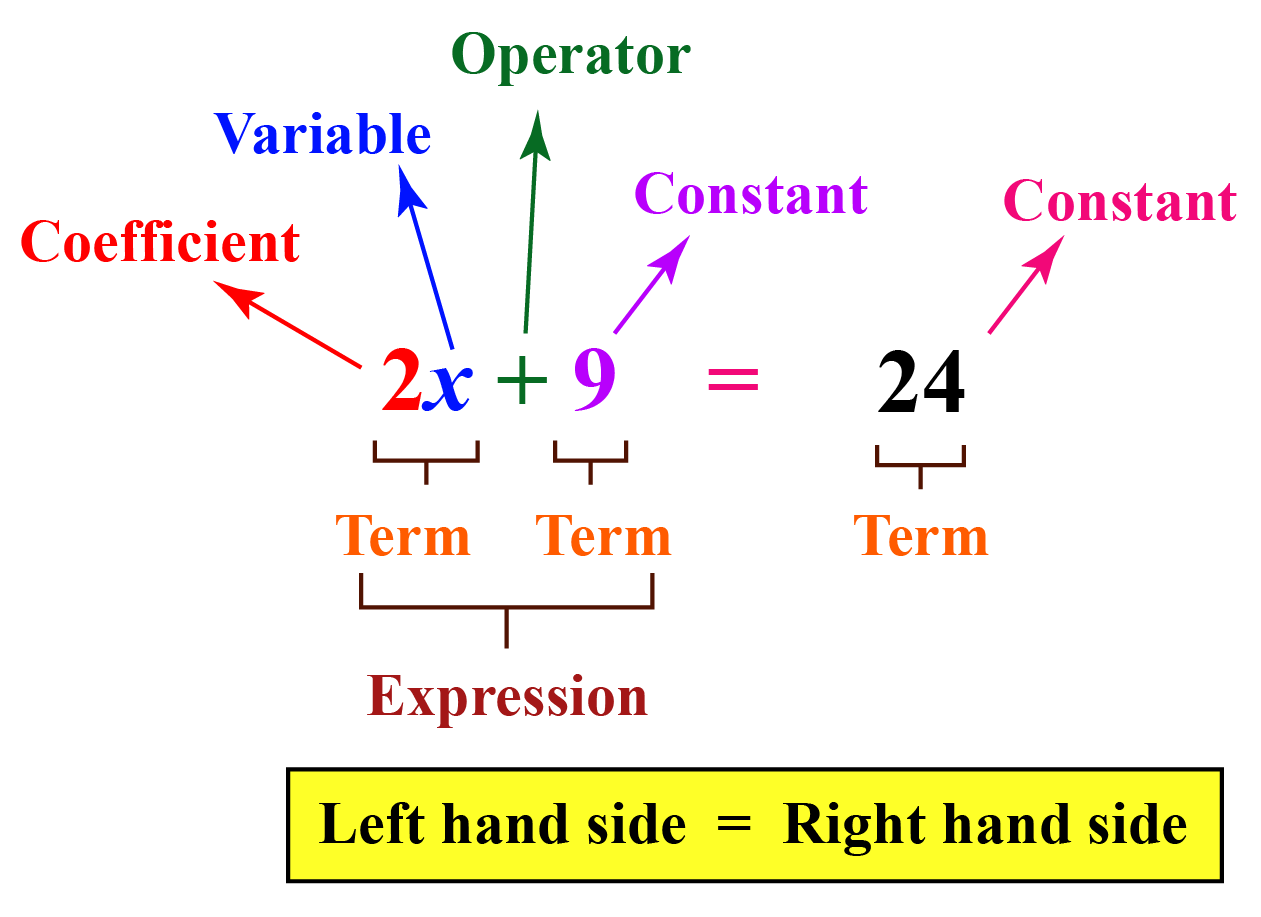
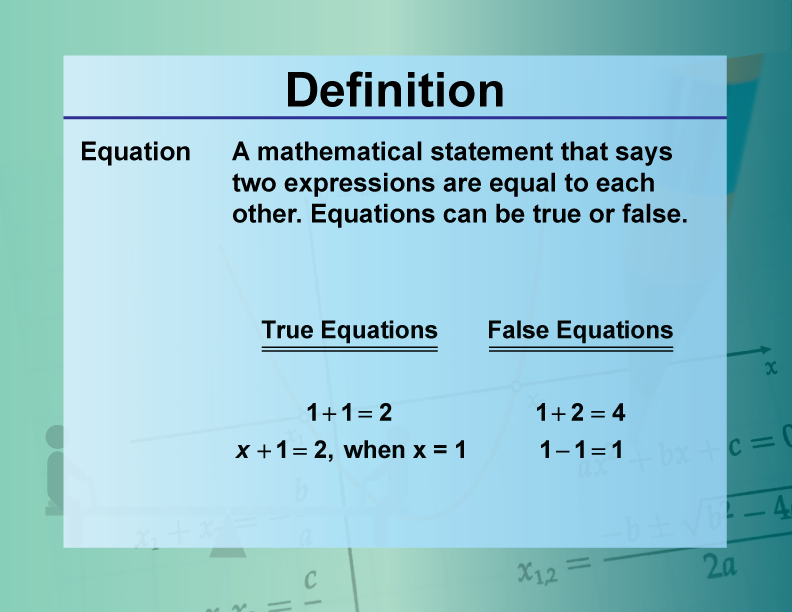
At its core, mathematics uses equations to describe relationships. An equation is, quite simply, a statement that two things are equal. This equality is represented by the ubiquitous equals sign (=). Think of it as a bridge connecting two different expressions, ensuring that what's on one side is balanced by what's on the other. This fundamental principle allows us to explore the intricacies of the mathematical universe.
| Category | Details |
|---|---|
| The Essence of an Equation | An equation in math is defined as an equality relationship existing between two expressions situated on either side of the equal to sign. |
| Illustrative Example | For instance, the expression 3y = 16 qualifies as an equation. |
| Variables Unveiled | Within the context of mathematical equations, a variable is frequently denoted by a letter. This letter serves to symbolize an unknown value, a placeholder until the specific number it represents is ascertained. |
| The Function of Mathematical Symbols | Mathematical symbols constitute the fundamental building blocks of all mathematical functions. |
| Linear Equations Explained | A linear equation is characterized by the fact that the highest power of the variable is always 1. Graphically, this results in a straight line, which explains the term 'linear'. |
| The Importance of Symbols in Math | Symbols play a crucial role in saving time and space when writing mathematical expressions. |
| Historical Significance of Symbols | The history of these symbols is varied. Some have been in use for centuries while others have been introduced more recently. |
| Examples of Equations and Symbols | An equation states that two things are equal. It will have an equals sign = like this: 7 + 2 = 10. The list below has some of the most common symbols in mathematics. |
| Multiple Meanings of Mathematical Symbols | Most symbols have multiple meanings that are generally distinguished either by the area of mathematics where they are used or by their syntax, that is, by their position inside a formula and the nature of the other parts of the formula that are close to them. |
| The Power of Equations | An equation in math is an equality relationship between two expressions written on both sides of the equal to sign. |
| Understanding the Mean (Average) | The mean (average) of a set of data is calculated by adding all numbers in the data set and then dividing by the number of values in the set. |
| The Median: The Middle Ground | When the data set is ordered from least to greatest, the median is the middle value. |
| Solving Complex Equations | There are special ways of solving some types of equations. Learn how to solve quadratic equations; Solve equations with sine, cosine and tangent. You should always check that your solution really is a solution. |
| Types of Equations Based on Degree | Based on the degree, equations can be classified into three types. Following are the three types of equations in math: Equations with 1 as the degree are known as linear equations in math. In such equations, 1 is the highest exponent of terms. |
| Understanding Subset notation | May mean that a is a proper subset of b, that is the two sets are different, and every element of a belongs to b; Expresseed as a formula, a b {\displaystyle a\subseteq b} ab means that a is a subset of b. |
| Math Bracket Overview | Math brackets are symbols used to group numbers and mathematical expressions together, giving these grouped numbers or expressions higher priority over other operations in the equation. What does a bracket look like? When we picture a bracket in math, we probably think of the round, symmetrical curves ( ). |
Consider the simple equation: 7 + 2 = 10 - 1. This equation expresses a truth: the sum of 7 and 2 is equivalent to the result of subtracting 1 from 10. This straightforward statement highlights the essence of an equation, that one side balances the other. The equation also demonstrates how simple arithmetic can form part of more extensive mathematical expressions.
- Calculate The Mean Formulas Examples Types Learn Now
- Britt Michael Are They Still Together Plus More Updates
Equations aren't just about numbers; they can also involve variables. A variable is a letter, such as 'x' or 'y,' that represents an unknown value. This element of uncertainty allows us to build more complex equations, where we seek to discover the hidden value of the variable. For instance, in the equation 3y = 16, the variable 'y' waits to be unveiled.
Let's delve into some fundamental concepts, starting with the mean, commonly known as the average. Calculating the mean involves adding all the numbers in a set and then dividing by the count of those numbers. This single value gives a central representation of the dataset, providing insight into the overall distribution of values. "Add up all the numbers, then divide by how many numbers there are. In other words it is the sum divided by the count."
There's more to the concept of 'mean' than just a basic average. Depending on the nature of the data or the research's objectives, different forms of 'mean' can be utilized. For instance, weighted means give specific data points more significance, depending on their contribution to the entire calculation. In other instances, values can be multiplied instead of added, or reciprocals can take their place.
Another concept is the median. The median is the middle value when data is arranged in order from least to greatest. It is a measure of central tendency, particularly useful when dealing with datasets where extreme values might distort the mean. For example, if a dataset includes a range of income, with some exceptionally high earners, the median income will provide a more representative picture of the typical income in the group than the mean (average) income. The mean can be influenced by outliers, while the median offers a more resistant value.
Now, let's turn our attention to mathematical symbols. Just as letters form words, mathematical symbols coalesce to form expressions and equations. These symbols serve as the building blocks, allowing mathematicians to express intricate concepts concisely. The list below has some of the most common symbols in mathematics.
Symbols such as the plus sign (+), minus sign (-), multiplication sign (), and division sign () are used daily. The equal sign (=), which signifies equality, and the various types of brackets parentheses ( ), brackets [ ], and braces { } are equally significant for expressing mathematical relationships. However, the use and the meanings of these symbols vary based on the area of mathematics where they are used or by their position inside a formula and the nature of the other parts of the formula that are close to them.
The symbols that are used in mathematics save time and space when writing, and they help encapsulate complex ideas in a compact form. They are also a fundamental element of a universal language, a language that transcends linguistic barriers. The history of these symbols is as varied as their meanings.
In set theory, the colon symbol is used to represent a "such that" statement. It is used in an expression such as this one, which defines the set of complex numbers. For example, the expression "x | x > 5" means "the set of all x such that x is greater than 5." Also, when two sets are being compared, like when defining that set a is a subset of b, this can be represented as "a b," which means that every element of a belongs to b, though there may be elements of b that are not in a.
Math brackets are symbols used to group numbers and mathematical expressions together, giving these grouped numbers or expressions higher priority over other operations in the equation. We probably think of the round, symmetrical curves ( ). They help with the order of operations.
The importance of PEMDAS (Parentheses, Exponents, Multiplication and Division, Addition and Subtraction) cannot be overstated in this context. This acronym acts as a memory aid, meticulously guiding you through the order in which operations should be executed to solve a mathematical problem effectively. The correct use of the PEMDAS rule ensures that equations are solved systematically, leading to accurate and reliable results. For example, when presented with an equation that involves multiple operations such as addition, multiplication and parenthesis, PEMDAS clearly defines the order to perform the operations, starting with the expressions within parenthesis, then evaluating exponents, then multiplication, then division, and finally, addition and subtraction. PEMDAS maintains consistency.
Equations can be classified into different types based on their degree. Equations with 1 as the degree are known as linear equations. In such equations, 1 is the highest exponent of terms. When this equation is graphed, it always results in a straight line. This is the reason why it is termed as a 'linear equation'. There are linear equations in one variable, in two variables, in three variables, and
In mathematics, the colon symbol has several uses, depending on the context. An example can be found when defining a set or in set theory: {x | x > 5}.
An equation is a mathematical statement that two things are equal. It consists of two expressions, one on each side of an 'equals' sign. This equation states that 12 is equal to the sum of 7 and 5, which is obviously true. For example: 7 + 2 = 10. Equations are statements that two things are equal.
In the specific case of the vertical line on a graph, we use the equation x = 1.5 for the line that has x coordinate 1.5. Every point on the line has x coordinate 1.5, that is why its equation is x = 1.5.
The concepts of parameters and variables are linked in certain mathematical contexts. Parameters often serve as constants within an equation, while variables are the values that can change. Quite often, the terms variables and parameters are used interchangeably, but with a semicolon the meaning is that we are defining a function of the parameters that returns a function of the variables. For example, the values (parameters) might change based on an experiment, but there can still be a variable within the function. When constructing a linear equation, one needs to choose values (parameters) that will be multiplied by a variable (the variable) to solve for a value.